A System for Measuring the Complex Microwave Magnetic Susceptibility ( Permeability) of Small Samples at High Temperatures
Details of the MPN Cavity Perturbation Technique :
A system has been developed, based on the cavity perturbation technique, for measuring the complex scalar permeability of materials at frequencies between 915 MHz and 2.8 GHz, and up to a temperature of 1200°C. The system is suitable for measurements on most classes of materials, including ferrites, advanced magnetic ceramics, fabrics, earthenwares, geological and biological materials and glasses. The system measures the complex permeability of materials during conventional heating, curing or sintering.
A hot sample and its holder are rapidly removed from a conventional furnace and inserted into a high magnetic field region of a thick-walled, cooled multimode cavity. The resonant frequency and loaded Q of the cavity are measured by a Hewlett-Packard 8753 network analyzer and stored for off-line analysis which includes subtraction of hot empty sample holder effects. The sample and holder are either left in the cavity for further measurements at lower temperatures as the sample cools, or are quickly returned to the furnace for further processing. In the latter case, the sample can be out of the furnace for as little as 2 seconds for a measurement at a single frequency.
The data analysis uses the general formalism of resonant cavity frequency shift theory, in which the real and imaginary parts of the complex magnetic susceptibility, χm= μr-1, are related, through functions dependent on sample shape, to the change in cavity resonant frequency and Q [1,2]. The absolute calibration is normally done at room temperatures, and uses measurements on known materials (similar to ASTM Standard Test Method, D2520-86, Method C).
Multimode Cavities for Multifrequency Analysis
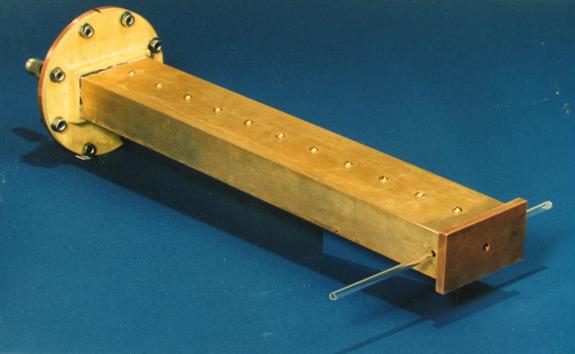
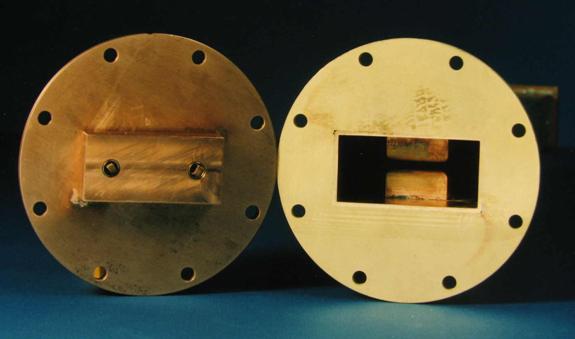
The peak-analysis and frequency-agile abilities of the HP 8753 network analyzer allow measurements of frequency and Q at eight frequencies in less than 16 seconds. To take advantage of this capability, a cavity is required with good higher-order-mode separation (at least to eighth order) and with relatively high magnetic field in the sample region. The resonator used is based on a shorted 50 cm long section of heavily ridge-loaded WR-284 waveguide, operating in the TE10n modes. The ridges terminate ~ 2cm before the flat metal plate short on each end of the waveguide, and the samples are inserted in the open end-region, transverse to the waveguide, with sample axis parallel to the wide (“b”) dimension. The sample geometry is a long, small-diameter cylinder ( 3.4:1 length/diameter ), oriented in the direction of the central (transverse) RF magnetic fields. The copper walls in the region of the gap are water cooled. All modes up to and including the eighth mode at 2800 MHz are cleanly separated from other resonant frequencies.
The Data Analysis Formula
μr = μr´ - j μr′′ = χm +1 = χm´ +1 - j χm′′ may be determined from resonant-cavity perturbation measurements using the following equation:
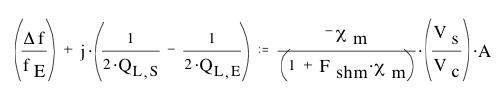
where fE, QL,E are the cavity frequency and loaded Q, with the empty holder,
- Δf is the frequency shift produced by the sample,
- QL,S is the loaded cavity Q, with the holder and sample,
- Fshm is a real number dependent only on the sample shape,
- Vs and Vc are the sample and cavity volumes,
- A is a real calibration constant dependent only on the shape of the magnetic fields in the absence of the sample. Note that when there is a small but significant electric field at the location of the sample, the contribution to Δf and (2*Q)-1 due to the known dielectric properties must first be subtracted before the magnetic properties are determined.
The two calibration constants in the formula, A and Fshm can be determined by comparison with known solid calibration samples. Note that both the real and absorptive parts of the susceptibility are given by Eqn. 1, even though the calibration constants are real and may be determined by measurements on low loss magnetic materials. For large μ′m, the fractional frequency shift reaches a limiting value which is independent of the susceptibility. As well, a metal sample without magnetic properties ( copper for example) still has the property of rejecting all electric and magnetic rf fields from its volume, which leads to the equivalent ( via Lenz`s law) of χm = -1 .
The cavity has a small but significant electric field in the region where the samples are placed especially at the higher frequencies, so the effects of the frequency shift caused by the dielectric response of the sample must be subtracted during the data analysis. To calibrate for the effect of the dielectric constant, sample materials with pure dielectric response ( ie., no magnetic properties ) are measured in the cavity at the magnetic measurement location, and after the aforementioned corrections are applied, they must yield zero magnetic susceptibility ( ie., χm = 0 ). The dielectric calibrators used are (1) single crystal, high-purity sapphire (εr´ = 9.35 with electric field perpendicular to the C-axis), (2) teflon (εr´ = 2.07) and (3) an ATC-90 low loss high dielectric constant material.
The magnetic susceptibility calibration is done by averaging over a few materials which have been measured extensively by other groups – in the present case, G 810 (Transtech), Ferrite 50, TT2-111R and also metallic copper ( susceptibility = -1 ). This technique was chosen because there are no invariant, well known materials with reproducible, (ie., independent of manufacturing technique) magnetic responses.
The overall absolute error of this magnetic susceptibility calibration technique for the present sample length to diameter ratio (~3.5) depends on the value of the dielectric constant, but for materials with εr < 20, was shown to be less than ± 20% . The relative values at different temperatures and frequencies should have errors less than 10%.
Sample Holder Considerations and Sample Preparation Methods
Rapid movement of the sample in and out of a high temperature furnace requires the sample holder to be relatively insensitive to temperature gradients and to high cooling and heating rates. High purity amorphous silica can tolerate these conditions as well as being inert in air up to 1600°C. Thus, at present, the sample holders for high temperature work are made from amorphous silica tubes, 1 mm wall thickness, and 6.0 and 4.0 mm inner diameter for the low and high frequency cavities, respectively. A 1 mm thick flat bottom is fused into the tube to support the sample. Teflon sample holders may be used for low temperature studies.
High purity, low OH content amorphous silica has εr´ = 3.8 and tan δ = 10-4 at room temperature. However at high temperatures (>1000C), both εr´ and the losses increase significantly, and their contribution to the cavity frequency and Q shift value must be included. Before a measurement with a sample, the empty holder frequency shift values are measured at 100°C intervals up to the maximum planned temperature. They are later used to subtract the sample holder dielectric component from the sample magnetic measurements
The use of the amorphous silica holder is limited by two effects: 1) the gradual nucleation and recrystallization to cristoballite above 1000°C, and 2) chemical and physical interaction with the sample, particularly if the sample contains mobile or reactive metal ions. The former effect results in heavy "frosting" of the surface of the tube and, although there is very little change in the dielectric properties, embrittlement and cracking of the holder will eventually occur. Holders have survived over two hours at 1500°C, with measurements every fifteen minutes.
Chemical interaction with the holder is a function of the number of physical contact points; thus pressed pellets of reactive material can often be measured with insignificant contamination of the holder, while powder packed in the holder, produces intolerable reaction. Samples are usually prepared either by diamond core drilling of solid material or by pressuring powders into self-standing pellets. Non-reactive materials may be used in powder form.
During the last two years we have made measurements on a broad selection of materials to give us practical experience with many different sample types. This has given us a better understanding of the abilities and limitations of the apparatus and technique, and has revealed a few areas of study to which the system is especially suited. One point in particular that constantly recurred was the variability in microwave properties from sample to sample, both in composites (due to lack of homogeneity) or in pressed compacts or powders (due to density variations). It became clear that studies of temperature dependence and particularly of frequency dependence would have better accuracy and be easier to interpret for mechanistic studies if all measurements could be done on a single sample. This is especially true of measurements intended to mimic a materials processing step (such as microwave heating and curing) where the sample property changes are inherently irreversible.
Data Presentation , Tanδm and Field Attenuation Length
The fundamental properties measured by the system are the real part of the relative permeability, represented by the symbol μ′ or μp, and the imaginary or absorptive part of the relative permeability, represented by μ′′ or μpp. Plots of these quantities versus temperature are the basic data generated by the present cavity measurement system.
Many customers are interested in, or require, secondary quantities which are derived from the values of the complex permeability and permittivity of a material :
- 1) the quantity normally called “tanδm” or the “ magnetic loss tangent” as a function of defined by
tanδm≡μ′′⁄μ′. - This quantity is an indicator of how well the magnetic properties of the material will cause microwave absorption, assuming the microwaves are inside the material! Plots of tanδm versus temperature can cover a few orders of magnitude for a large temperature range , and are sometime presented as semi-log plots.
- 2) the TEM mode field attenuation length for a TEM wave propagating in a material with negligible dielectric loss.
The following yields the field attenuation constant, α ( in nepers/unit length ), for the situation of a TEM mode propagating in the z direction in the material with low dielectric loss. The inverse of α (ie. α-1 ) is called the field attenuation length, and is given by the following equation, where f is in MHz , and ε′ is the relative (ie. unitless) value of the real part of the dielectric constant (ε′′ is assumed zero), and μ′ and μ′′ are the components of the relative permeability:
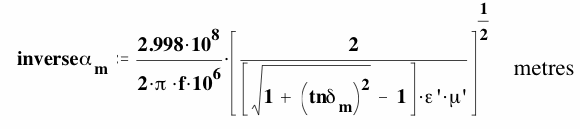
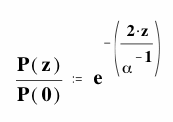
The power carried by the fields decreases as a function of depth ( power is proportional to E2 ), and the formula shown above gives the power carried by the wave as a function of depth. When the wave penetrates a distance equal to the inverse of the attenuation constant , the power in the wave is decreased to 13.5% of its initial value . The rate of power deposition in the material per unit depth is then proportional to
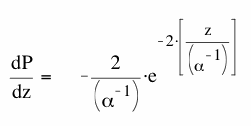
When the value of tanδmag is very large (and the value of ε″ is zero) , the expression for α-1 reduces to
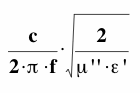
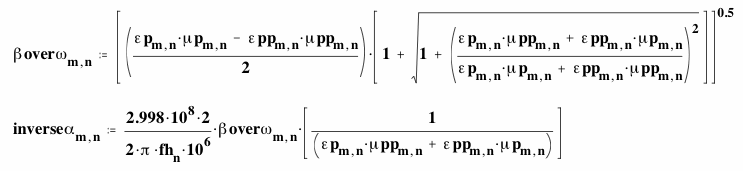
- 3) the TEM mode field attenuation length ( inverse α ) for a TEM wave propagating in a material with both dielectric and magnetic loss is given using the following expressions:


Please feel free to phone or e-mail and discuss possible measurements. We treat all communications as confidential unless advised otherwise.
